Analytic continuation

In analysis is meant by the analytical continuation of a function that is defined on a subset of the real or complex numbers, an analytic function, comprising on a complex area is defined and corresponds to the portion of the original function. Here are almost all the cases of interest in which the continuation ( and usually also a maximum area ) is uniquely determined by the predetermined amount and defined on their function.

In the theory of functions, in particular in studies of functions in several complex variables, the term is taken abstract. Here, analytic continuation resuming a holomorphic function and a holomorphic function germ. A distinction is made between the continuation of the germ along a path and the continuation to a function in a field. It is significant that holomorphic functions - can already be made ​​with local data on a very small area very well reconstructed - unlike, say, steady or only infinitely differentiable functions.

• 6.1 Existence and Uniqueness
• 6.2 Example

Analytic continuation in the Analysis

For the elementary calculus important statements about continuability are the following:

• Be a real (open or closed ) interval. Then a function is exactly then analytically continued,

• If there exists an open neighborhood of each point of the interval on which the function can be represented by an absolutely convergent power series, or
• If at any point of the interval is infinitely differentiable and the Taylor series at any point of the interval has a non-vanishing radius of convergence.

• If the closed hull of an infinite set coherently, so for example, is a real interval and an analytic continuation of a field exists, then agrees to a second holomorphic function already then with the continuation agreement,

• If it matches on an infinite subset of which accumulates in or
• If match in any fixed point of the function values ​​and all derivatives of and.

The mentioned here and some other theorems on analytic continuation and the uniqueness of the continuation are included in subsequent, more abstract formulations of the theory of functions as special cases.

Examples

• Every rational integral to function, so every real function whose function term is a polynomial in, lets continue to analytically by the function with the same function term.
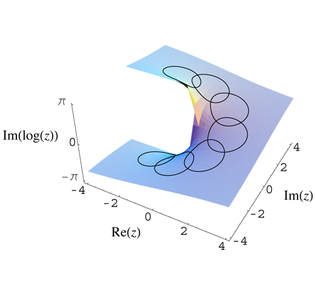
• The rational function can be continued to the area. Inside the unit circle, the continuation can be represented by the power series, the exterior through the Laurent series. Both sequels can be analytically continued locally by power series over their region of convergence beyond. They can thus be assembled into a common analytic continuation of this is, as always with fractional rational real functions, of course, the complex rational function.
• The real exponential functions, the sine function and the cosine function can be represented as a power series with radius of convergence. Therefore they can be analytically continue to all functions, which can then be represented by the same power series.
• The faculty to defined function has analytic continuation as the gamma function this sequel, however, is clearly only by the additional condition that the sequel should be logarithmically convex. → See the set of Bohr - Mollerup.

Germ

To give a precise definition of an analytic continuation in the sense of the theory of functions, the terms blade and function germ must first be explained: Let be a complex manifold and a point. In addition are two environments and two holomorphic functions. The two functions are called equivalent in point, if one exists around with. The set of all these equivalence classes is called the stalk, the equivalence classes as (function ) germs. The projection of a function on their germ in point is quoted at.

Clearly, the germ of a function describes the behavior of " immediate " in around. This is more than the mere function value, because the discharges etc. can be read from the seed, as they result from even the smallest around.

The blade carries a natural way the structure of an algebra. It is isomorphic to the algebra, since the local behavior of a holomorphic function is uniquely determined by its power series expansion in convergent power series.

Continuation along a path

Be a contiguous complex manifold, two points and two function germs. is called analytic continuation of along the way with if the following holds: There exist points with open environments and holomorphic functions such that

In other words, there is a finite sequence of open environments, which overlap the curve. Each holomorphic functions are defined in those environments which are identical in the regions where they overlap environments. Often one chooses open circles as quantities, because these appear as areas of convergence of series expansions; in this case one speaks of a circular chain.

This continuation generally depends on the choice of the path from (but not of the intermediate points and the surroundings ). Also, there is generally no in an environment of very holomorphic function with and.

Definition

Be a contiguous complex manifold, a point and a function germ. The quadruple is called an analytic continuation of, if the following applies:

• Is a connected complex manifold.
• Is a holomorphic map and a local homeomorphism.
• Is a holomorphic function.
• So that and, where the projection of the equivalence class referred to in their seed.

The thus defined, analytic continuation is related to the continuation along a path: If a path with start point and end point, then is a way to begin and end points. The function defined in a neighborhood of by a function germ.

Example

And be the germ in that branch of the holomorphic square root. Analytic continuations of these are for example:

• Defined by the Taylor series to the open disk function. The projection is the natural inclusion mapping.
• The square root of the main branch, defined on the complex plane of the slotted, again is the natural inclusion mapping.
• The square root of the main branch, defined on the complex plane of the slotted, again is the natural inclusion mapping.

All examples have in common that can be considered as a subset of. The last two examples also show that there is no within the largest area in which the function can be continued holomorphically. The question of the maximum continuation leads to the definition of the maximum analytic continuation:

Maximum analytic continuation

Be a contiguous complex manifold, a point and a function germ. An analytic continuation of analytic continuation is called maximal if for any other valid by analytic continuation: There exists a holomorphic map with, and.

Existence and uniqueness

Directly from the definition it follows the uniqueness of the maximal analytic continuation up to holomorphic isomorphism. The existence can be shown by using the sheaf theory is the connected component of the overlay area of ​​sheaf of holomorphic functions, which includes a fixed chosen archetype of the germ.

Example

And was the germ of that branch of the holomorphic square root. The maximum analytic continuation is given by:

At another analytic continuation is defined by the mapping.