Attractor#Strange attractor

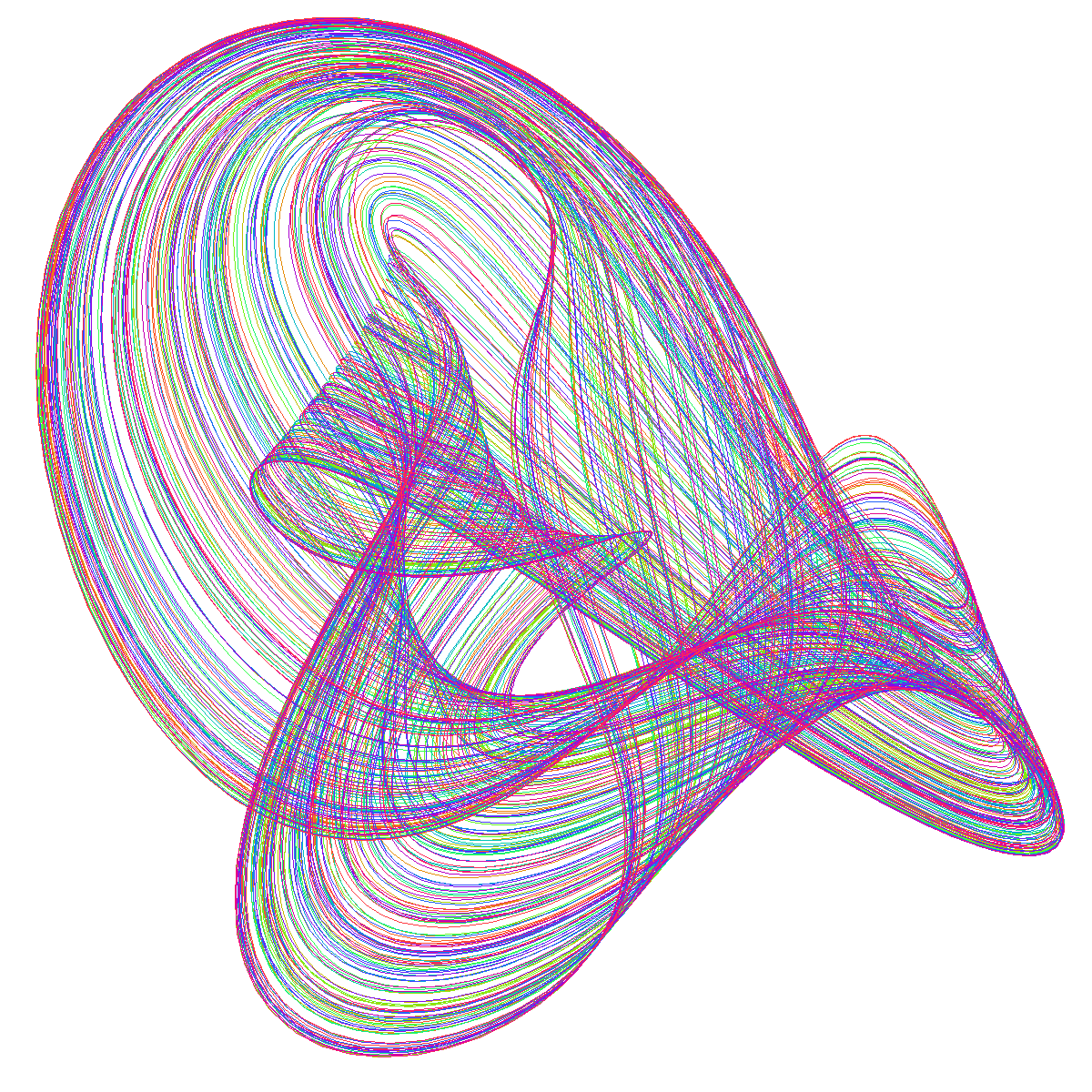
A Strange attractor is an attractor, ie a place in the phase space, which represents the final state of a dynamic process whose dimension is not an integer. It thus is a fractal that can not be described geometrically in closed form. Occasionally, the term is Chaotic attractor preferred because the " strangeness " of this object can be explained using the tools of chaos theory. The dynamic process shows an aperiodic behavior.

• 5.1 box dimension
• 5.2 Information Dimension
• 5.3 Lyapunov dimension

Discovery

The term Strangely attractor can be traced back to an article by David Ruelle and Floris Takens in 1971, who had the mathematical background of the formation of turbulent flows on the topic. Since 1963, the Lorenz attractor was known, a mathematical structure that was discovered in the modeling of air flows under the influence of temperature differences.

Attractors have been studied previously for the description of dynamic processes. However, this is usually presented as a classical geometric structures before, such as points or cyclically traversed lines. Acyclic attractors was known, too, but kept them for special cases, anomalies that can occur only with a specific choice of parameters.

With the introduction of the concept of strange attractor, it was possible to better understand the legalities of chaotic behavior in dynamical systems and to describe quantitatively. This behavior, such as turbulent flows of liquids and gases, which is characterized by a lack of periodicity and sensitive dependence on initial conditions ( butterfly effect ), previously eluded by its complexity of an analytical approach. With designs such as the strange attractors it is possible to describe a deterministic, but not predictable behavior ( deterministic chaos) mathematically.

Definition

From a strange attractor occurs when the following conditions are met:

• It is an attractor in a dynamic system with a specific catchment area
• Chaotic behavior: Any small changes in the initial state lead to completely different courses
• Fractal structure: The attractor has a non -integer dimension
• No splitting option: Each track that starts in the catchment area, approaching any heavily on each point of the attractor.

Examples

Some classic examples, the properties of strange attractors can be studied quite well. The dynamic system used may be discrete or continuous. Continuous systems are usually described by differential equations in the phase space, these systems form an outgoing line from the initial state, the trajectory. Because of the uniqueness of the derivative itself trajectories may intersect at any point. This result suggests that attractors can only have a simple structure in two dimensions, strange attractors, and thus chaotic systems are in continuous dynamic systems only in a phase space with at least three dimensions.

Hénon attractor

A relatively simple example of a strange attractor of the Hénon attractor is (named after Michel Hénon ), which is defined as a discrete system in two-dimensional space by the following equations:

Each imaging step can be divided into three steps disassemble: A folding - stretching operation by adding to a contraction by a factor along the axis and a mirror under the exchange of the - and -axis. In the choice of parameters and the typical image of the Hénon attractor results if one follows the path of a start point, which is sufficiently close to the attractor. Superficially looks like a line of the attractor, which is placed in a few wrinkles, however, since it is invariant to the above-described folding mirror - stretch-shrink operation, each line is again divided into endlessly approximately parallel lines. A cross section through the attractor has the structure of a Cantor set, so the attractor has a fractal structure.

Considering the environment of a point on the attractor, that is a circular disk with a small diameter, it will be converted by a mapping step in an elongated ellipse that is elongated along the lines of the attractor, by the contraction step but has a lower surface. With continued application of the mapping rule, the image of the point environment covered ever larger areas of the attractor, while its surface tends to zero.

Rössler attractor

The Rössler attractor ( in 1976 by ​​Otto Rössler discovered ) is defined by the following system of differential equations in three-dimensional space:

The Rössler attractor has been defined in order to study the phenomena of time already longer known Lorenz attractor for a simpler example. The dynamics of the underlying system can be illustrated as follows: In general, the trajectories run in the xy plane in a circular orbit counterclockwise around the origin and form a fixed -limited flat band. The band broadens as it extends in the range of high values ​​increase the trajectories in z- direction, whereby they are guided to the inside of the circular path. The outer portion of the belt is folded to a certain extent inside. As indicated above, trajectories can not intersect, the band thus consists of two closely superposed layers that approximate more and more, but still have a distance from each other. For each subsequent pass of the circular path, the tape is folded once again, it is thus an infinite number of layers, which are as close to each other. A cross-section through these layers again yields a Cantor set.

Again, the fractal structure is produced by an infinite sequence of stretching and folding operations. Looking at the trajectories of two close points, they will be a time run long relatively close to each other, but by the stretching increasingly diverge until once the point is reached that a trajectory on the flat part goes, the others are on the above folded. In order for a connection between the two trajectories is no longer given, we are dealing with chaotic behavior.

Lorenz attractor

The Lorenz attractor was discovered in 1963 by Edward N. Lorenz in the modeling of air flows. Defined by the following equation system:

The three parameters are determined by the underlying physical model and Lorenz were given with and.

The attractor is symmetrical about the axis and is composed of two disc-like parts which are slightly tilted towards the axis. Here are the trajectories, as in the Rössler attractor, flat circular bands which widen when running around the respective circle. The direction of rotation is at the bottom of the "slices" to the outside towards the center, the next from top bands approaching more and more and then lie flat on each other. One half of the tape after forming the tape of the one and the other of the other circle. So it's here in continued stretching folding part operation. A trajectory can be one or more rounds in one, then one or more remain in the other subsystem, its number is virtually unlimited and depends on the initial value. Again trajectories are closely spaced points for a certain amount of time to run parallel and do the same Kreisumläufe up in the division, a trajectory on the one and the other ends up on the other side and thus a completely different further outcome occurs.

Lyapunov exponent

In order to describe the behavior of a dynamic system quantitatively, the Lyapunov exponents are usually used. These describe the dynamic behavior of the environment of a point on the attractor: First, it is expected that a point of interest is attracted by the attractor, which is expressed by a negative Lyapunov exponent whose magnitude is a measure of the strength of attraction. If it is a strange attractor, a repulsion of closely spaced points, as seen in the examples observed, which corresponds to a positive Lyapunov exponent. In fact, the behavior is dependent on the direction, the two points to each other. If you look at the neighborhood of a point as a circular disc or ball before, so this is deformed in the course to a narrowed and elongated image. To reflect this, a dynamic system has as many Lyapunov exponents as dimensions of the phase space.

Performs one calculation steps for a point on the attractor and a different point in its vicinity, the first Lyapunov exponent by

Defined. is the deviation in the calculation step, so it is the average error gain is determined for large.

The first Lyapunov exponent is always the value of the largest error gain, ie, the strongest repulsion. This is achieved by determining the threshold for: For any choice of the perturbed starting point, the direction of deviation is generally a combination of a fault in the direction of greatest error gain and other disorders with lower gain. One keeps in further calculation steps, the direction of the disturbance at predominates after several steps more and more the biggest mistake reinforcement.

In the numerical calculation of the first Lyapunov exponent, provision must be made to actually execute any number of steps can be: After each step, a renormalization is performed, ie, the newly calculated point is disturbed before the next step is replaced by a point, from the undisturbed point has the same direction, but the same distance as before the computation step. Thereby it is avoided that the gain of the initial error reaches a magnitude that distorts in the geometrical characteristics of the attractor, which already has a finite size, the result.

The remaining Lyapunov exponents are defined analogously: Is determined by the maximum distance change, it follows from the maximum change in area in the vicinity of a Attraktorpunktes, from the maximum change of space and so on. Often the other Lyapunov exponents can be calculated by means of the first, as can be from the definition of the dynamic system are derived the factors of area and space contraction. In an attractor the sum of all Lyapunov exponents is generally negative, with a strange attractor but is at least the first positive.

Dimension

An important indicator of a fractal, and thus for a strange attractor, is the dimension. There are several ways to extend the notion of dimension that can take on only integer values ​​in the classical geometry on fractals. Necessarily, all these definitions in classical geometric objects give rise to their well-known dimension, so for example, 1 for lines and 2 for surfaces. For a fractal different definitions of fractal dimension can but certainly result in different values ​​.

Box dimension

Most often, the box dimension is used for fractals. The basic idea is the subdivision of the surrounding space into equal-sized room elements ( "boxes" ), whose side length is reduced at each step. It is counted in how many elements of this room is a part of the Fractal. For the side length s is this number N ( s ) would be expected, then the following relationship: . is the dimension of the Fractal. Does this example, the value is 1, then the number of space elements in which is a part of the investigated structure, proportional to the reciprocal of the side length, which is exactly what one would expect for linear structures. By reducing the side length of the examined fractal is shown always accurate, so that one expects an increasing accuracy.

For the calculation of the dimension of a strange attractor, this method proves to but as not very helpful. The smaller the page size, the more space elements come into consideration, it must be made very many calculation steps of the attractor, without knowing whether all space elements are already recorded, in which the attractor lies. Especially in the size ranges that should provide increasingly more accurate values ​​, the calculation error increases.

Information dimension

To get the problems with the box dimension at least partially into the handle, you can refine this notion of dimension slightly. Counts in the box dimension only, whether in a space element at all is a part of the fractal, the size of this share is now at first (it's not particularly useful to speak of area or volume content ) was determined. In the case of the strange attractor That can be easily done by counting the iterations whose end point lies in the relevant space element. This portion (a number between 0 and 1) is referred to as a natural level. The information measured in bits, that a certain point of the attractor is in a certain space element is calculated as a binary logarithm of the reciprocal of the natural degree, the lower the proportion of the attractor, which is in this area element, the greater the information if the examined point lies.

The dimension information is obtained by the logarithm is replaced by the information of the entire system in the determination of the box dimension:

This is the average of the information for the individual spatial elements, weighted according to their natural level, or the average of the information of all the calculated points of the attractor.

Space elements that are not detected until very late in the course of the calculation as part of the attractor, also contain only a small proportion of the attractor and provide little to the overall information of the system. Thus, the calculation error is greatly reduced by early termination of the calculation, contrary to what the box dimension.

The information dimension is not always equal to the box dimension, it is the inequality.

Lyapunov dimension

Another concept of dimension is based on the conjecture of Kaplan- Yorke. This conjecture asserts that the information dimension is identical to the Lyapunov dimension, a size that can easily be calculated from the relative Lyapunov exponent. To determine these Lyapunov dimension to draw in a coordinate system on each n the value and connecting the points with straight lines. In a strange attractor of the first Lyapunov exponent is positive, the sum of all Lyapunov exponents is negative, so that cuts a piece of this polyline, the x -axis. The x value of this intersection is the Lyapunov dimension. In the case of a fixed-point attractor all Lyapunov exponents are negative, the intersection with the x - axis is therefore in the origin, the Lyapunov dimension is 0 with a cyclic, linear attractor is, all other Lyapunov exponents are negative. Here is the intersection of the x - value 1 so that is also confirmed in this classic case, the correctness of the Lyapunov dimension.

The meaning of the Lyapunov dimension lies in the possibility of their numerical calculation. While the determination of the box and the information dimension is nearing especially in higher-dimensional phase spaces to their limits, the determination of Lyapunov exponents and thus the Lyapunov dimension is still often possible.