Bandwidth (signal processing)

The bandwidth is a parameter in the signal processing that specifies the width of the interval in a frequency range in which are the dominant frequency components of a to be transmitted or stored signal. The bandwidth is characterized by a lower and an upper limit frequency, depending on the application requirements of the two different threshold values ​​, and thus, there exist different bandwidths as a characteristic value depending on the context. The term used to describe signal transmission systems in various fields such as communications technology, wireless technology or acoustics.

Definitions

Each transmission channel has - depending on its physical properties - a lower and an upper limit frequency. The lower frequency limit can be zero; in this case one speaks of baseband, otherwise bandpass location. The amount of difference between the two cutoff frequency values ​​is called bandwidth. The cutoff frequencies are either in the unit Hertz (Hz) and usually abbreviated f or designated by the angular frequency in units of s -1 and as.

For the determination of the cut-off frequencies and thus the bandwidth of different definitions are used depending on the application and reference. These different definitions can, with identical physical properties, lead to different bandwidth information. Below are some common definitions of bandwidth are described.

Strict band limitation

A signal is then band limited strictly, if the magnitude frequency response, with the parameter as the angular frequency, is outside the range of the bandwidth is equal to 0. This is real only in approximation possible and the nature of the bandwidth definition used in the context of signal theory as a simplified model.

For baseband signals with strict band limitation, the bandwidth is limited by an ideal low-pass. Real signals at baseband always exhibit negative frequency components, so-called mirror spectrum, as exemplified in the illustration on the magnitude frequency curve of a real-valued signal. The bandwidth is without the negative frequency component set to:

For signals in a so-called band-pass position, the band limitation is performed by a bandpass filter. Signals in bandpass situation arise, for example by modulating a baseband signal, they only compete in intermediate frequency stages in radios. By the modulation, the center frequency of the baseband signal is shifted from zero to the carrier frequency, thus the lower cutoff frequency has a positive value:

Negative spectral components, as in the baseband, not counting the added bandwidth. It should be noted that the reflection spectrum of the real-valued baseband signal through the frequency shift with a linear modulation to a doubling of bandwidth in the band-pass position, since the negative frequencies are shifted by the modulation in the positive spectral range:

Below the two positive partial spectra and above the center frequency are designated as lower and upper side band and contribute to real-valued base -band signals have the same information content. For the baseband signals, which have no negative frequencies, this is the case with an analytical signal, the bandwidth of both the baseband and in bandpass position is identical - analytical signals can be present only in the base band as a complex signal. Technically, this property is implemented in different ways, such as with the single sideband.

In non-linear modulation techniques such as frequency modulation, there is no direct relationship between the bandwidth of the baseband signal and the required bandwidth in the band pass area. The bandwidth is thereby expressed approximately by the frequency deviation in the Carson formula.

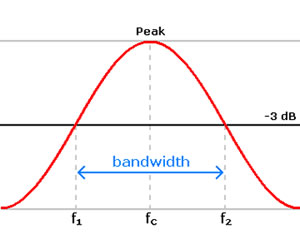
In real systems are due to the only finite attenuation of filters spectral spread over the entire spectrum, with a strict definition of the characteristic size of the bandwidth would be infinitely large and thus not very meaningful. As a practical characteristic value, the 3- dB bandwidth is usual, which is defined by the power density spectrum of the magnitude maximum. The cut-off frequencies are set at half maximum power value, which corresponds to a reduction to rounded 3 dB:

At the cutoff frequency, this corresponds to a reduction in amplitude by a factor.

The bandwidth is therefore at baseband to

And band-pass position

Determined.

For example, in the system corresponds to a low-pass filter, the 3- dB bandwidth of just the bandwidth of the filter, it is therefore also referred to as a 3- dB cutoff frequency.

In a series or parallel resonant circuit, the dimensionless relative bandwidth refers to the ratio of the 3- dB bandwidth and the center frequency:

The relative bandwidth is identical to the loss factor and the quality factor Q. reciprocally

Nyquist bandwidth

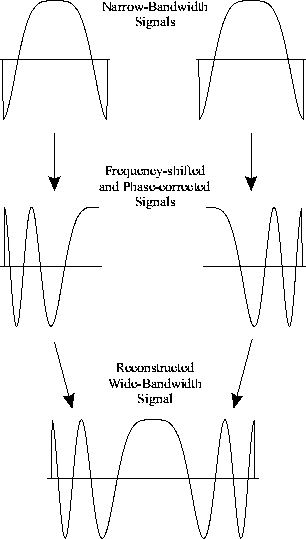
In theory, digital signal processing, the Nyquist -Shannon sampling theorem has a central position. It states that a continuous-time signal can be reconstructed exactly from any of the sampled discrete-time sequence, if the bandwidth of the signal maximum is half the sampling frequency. This maximum is referred to as the Nyquist bandwidth.

The naive reconstruction as a step function is indeed roughly: The square-wave pulses, the time strung together make up the staircase function, have a spectrum the sinc function, ie, infinite bandwidth. But the bandwidth within the first two zeros of the sinc function (for positive and negative frequencies) is just the Nyquist bandwidth. The product of the sampling period is 1, see time -bandwidth product.

Antenna Technology

In the field of antenna technology are relative, among other things, that dimensionless bandwidth data used. For narrowband antennas, these are antennas whose magnitude frequency response is approximately constant, a percentage bandwidth specification is used:

The theoretical maximum value of the percentage bandwidth is 200%, when the lower cut-off frequency is zero.

For broadband antennas, the magnitude frequency response is not constant, be related and expressed in the form as relative bandwidth specification, the two cut-off frequencies of the antenna:

Examples of bandwidth

The transmission system comprises a telephone in a first approximation to a lower limit frequency of 300 Hz and an upper cutoff frequency of 3400 Hz, which corresponds to a bandwidth of 3100 Hz, and sufficient for transmission of intelligible voice. Frequency components in the language, which will be below or above the cut-off frequency is suppressed in a telephone system by means of band-limiting and does not transmitted.