Square One (puzzle)

The Back to Square One ( Square One and Cube 21 or ) is a cube-shaped puzzle. By the way it is put together, it changes by twisting its shape and is no longer cubic. The object of the game is to get the game from any form of back in the cube-shaped state, using all colors to sort to the correct positions.

History

The Square One was invented in the 90s by Karel Hršel and Vojtěch Kopsky. They submitted on November 8, 1990, a patent for their patience.

The name probably comes from the English phrase "Back to Square One ," which means something like " Back to top ". As the cube was sold at the beginning of the twisted state, the name was quite appropriate.

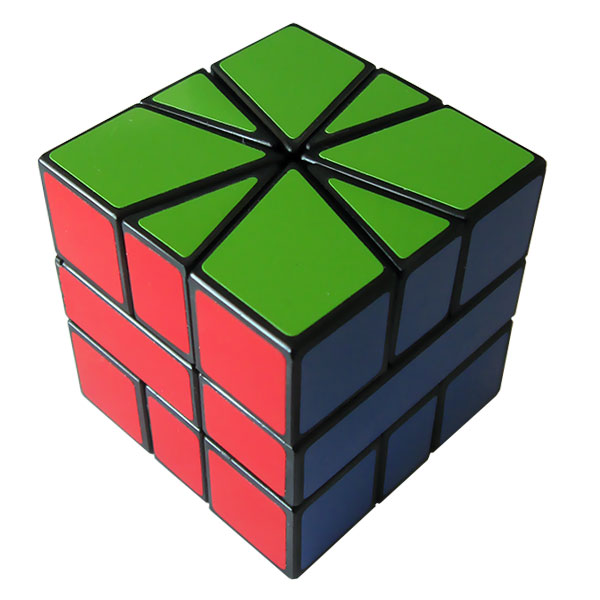
Construction

The Square One, consisting of three layers, has a remarkably simple structure. The top and bottom layers are each composed of four prisms with an equilateral triangle as base side - the edges of stones - and four prisms deltoids - the cornerstones. The middle layer is composed of two trapezoidal prisms, which together form a square prism. The corner and edge pieces can be easily eingehakelt in the two rails of the middle class. Once there is no stone, but also a gap between two stones is over the limit of 2 stones means you can turn the left or right side by 180 °. It can rotate more or less than 180 ° and the sides, but can be subsequently either the upper or middle or rotate the lower layer. From the position of Square One in the first image of this article, therefore, no horizontal plane can be rotated.

By the mechanism, it is possible to rotate the puzzle in any direction, allowing the parts. By this construction and mechanics, it is possible that the cube changes its shape and is not cubic.

In addition, each page in the dissolved state, similar to the Rubik 's Cube, its own color.

2 edges of stones

Two cornerstones

Mesolithic

Solutions

There are various solution approaches to Square One. One is to make the puzzle first cubical and then sort it color correct. Another approach is the layer -by-layer method, in which one is transferred from top to bottom, all the layers in the dissolved state.

The problem in solving the Square One is that algorithms are possibly not executable in a state of puzzle game, as we are changing through the twists of the possible axes of rotation.

Since the Square One is also achieved at official Speedcubing competitions - officially, that is by the World Cube Association WCA short, organized - there is also an official world record, this is 8.65 seconds, held by Bingliang Li from China.

Mathematical properties

Possible positions

Since the Square -1 8 edge stones and 8 cornerstones has, is angle at the top of curb stones by 30 ° and that of the cornerstones of 60 °, can the Back to Square One, if you let the middle level aside, assume 170 different forms. This is due to the fact that each level can ( except the middle ) consist of either 2, 3, 4, 5 or 6 cornerstones. 1 Eckstein is impossible, since otherwise you would need 10 edges to get to a sum of 360 °. 7 or all 8 edges stones are also not possible because at 6 corners and edges of the 0 level is already "full". Now there is one possible position when the level of a 6 cornerstones is (every 6 corners side by side). 3 positions at 5 cornerstones, each with 10 positions at 4 and at 3 and 5 positions cornerstones in a corner. The 170 positions can be calculated from the fact that if the first level has six cornerstones, the third logically must have two cornerstones, it follow 1 × 5 = 5 positions. Now, the first level may have 5 cornerstones, and the third plane 3 quoins and so on. Thus we come to the invoice

(1 x 5 ) (3 × 10 ) ( 10 × 10 ) ( 10 x 3) (5 × 1) = 170

Now there are, however, the average level. This can have 2 positions (square and concave quadrangle ). Therefore you have the 170 or multiply by 2.

Since the corner and edge pieces of the Square -1 not all have the same color, you have the 170 × 2 or 2 times with 8! multiply. This results in 170 × 2 × 8! × 8! = 552 738 816 000 positions.

However, this is only a preliminary result, as some of these positions, which were counted separately here are the same. Therefore, you have to deduct these positions again and comes to the final result of 435 891 456 000 possible positions or in other words, exactly 15! ÷ 3

God's algorithm

2005 Mike Mason Jones calculated the algorithm of God, ie the number of trains that is needed at least to the puzzle from every possible situation to solve, for the Square One. As was the case other magic cubes, this number could be dealt with on trains only by brute force, so it has been for every position of the shortest solution determined individually. Because this method used a computer was used to calculate used. It had a frequency of 800 MHz, and required about a year. The result was that every position can be solved with a maximum of 13 trains ( see table), even just 12 when not taken into account the average level.