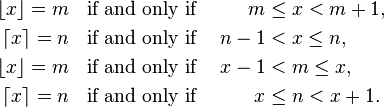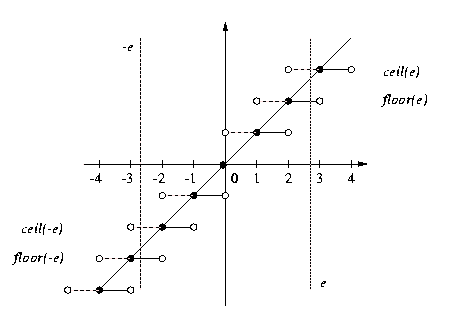Floor and ceiling functions

The rounding function (also floor function, integer, function, integer part function or Entier - clip) and the ceiling function are functions that assign a real number, the nearest not larger or smaller non- integer. The notation was named after Carl Friedrich Gauss, who introduced the symbol for the Floor function 1808. End of the 20th century, also introduced by Kenneth E. Iverson and designations ( engl. floor " floor ") for the floor function as well and ( engl. ceiling " ceiling ") for the ceiling function spread.

• 2.1 Definition
• 2.2 Examples
• 2.3 Properties

• 3.1 floor function and decimals
• 3.2 correlations between up and rounding function
• 3.3 Standard rounding

Rounding function or floor function

Definition

It is defined as follows:

Examples

Properties

• For all.

• It always works. It is precisely when an integer.

• For any integer and any real number.

• For all real numbers.

• For each integer and each natural number.

• The rounding function is idempotent: it is.

• If and are relatively prime natural numbers, then applies.

• The rounding function is not continuous, but steadily above.

• For non-integer real converges the Fourier series of the periodic function, and it is.

• If and so applies

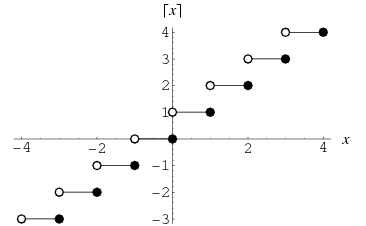
Ceiling function

Definition

It is defined as follows:

Examples

Properties

• It is analogous

• If and so applies

Main Features

Floor function and decimals

It applies to positive numbers:

Correlations between up and rounding function

• It is always

• For integers, the following applies:

Standard rounding

The rounded to the nearest whole number can also be expressed with these functions: